There is a very simple rule in life: Extreme returns only come from taking inordinate amounts of volatility.
I will explain this rule in the simplest terms and then provide a complex example.
Simple:
Let’s say you work a W2 salary job. Your income is fixed with zero volatility. You could always get fired but there isn’t any volatility in your income where one month you can paid less and the next month you get paid more.
The world isn’t this stable though. The world is chaotic, dangerous, and always shifting. Who is taking this volatility then? The equity holder/owner of the company that employs you. Now does taking volatility mean you will have exceptional returns? No. But exceptional returns can only be achieved through taking volatility. Volatility isn’t the goal or causal factor to exceptional returns but it is a necessary condition.
You have exposure to a higher payout than a W2 by simply being an equity holder. However, you have to take greater amounts of volatility. You get compensated for this volatility with the possibility of greater upside. There is no guarantee of greater upside though which means you might take volatility and NOT get an extreme payout. This means you have to deal with the swings AND uncertainty of not being paid. An employee with a salary doesn’t deal with any of this.
This is why you get paid to take risks. For exposure to an extreme payout, you need to have extreme mental fortitude.
Complex:
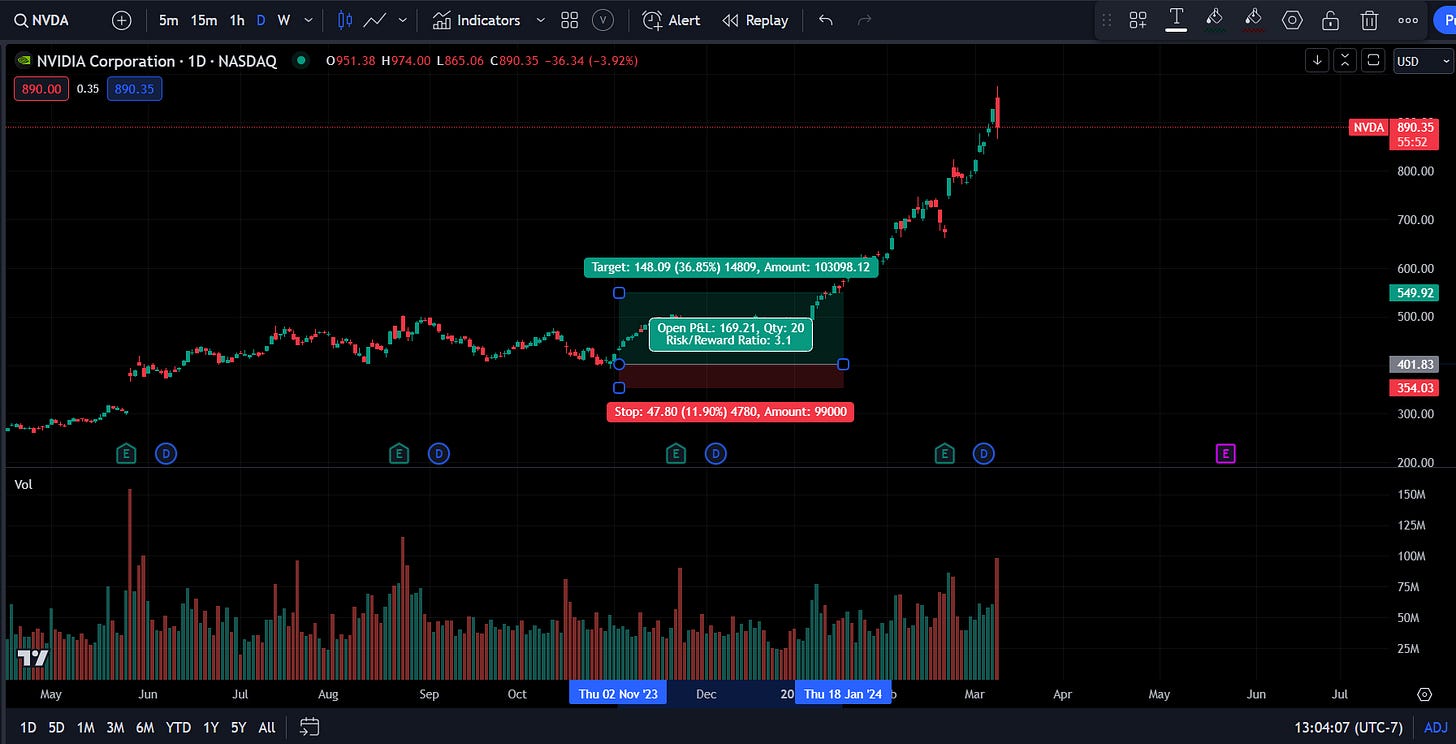
Let’s say last year you wanted to get long NVDA 0.00%↑ last year at 401.83 with stops at 354.03. Now let’s say you have a 100k portfolio and want to risk 1% ($1,000). This would mean buying 20 shares. Ok so you buy 20 shares ($8,036 notional) and NVDA moves up to your profit target of 549.92. If you didn’t run any other trades in your portfolio, you would end with a $3,000 profit and a notional position of $10,998. The total portfolio value would be around $103,000.
Now you face a choice. You have a much bigger notional position in the stock than you did previously. This means the swings will have a larger impact on your portfolio.
Choice 1: Move stops to breakeven to protect the original $1,000 you risked for the trade and let the position ride.
Choice 2: Trim the position marginally so that it takes up less notional size in your portfolio because you don’t want to take large swings.
If I go with Choice 1, I am basically accepting that if NVDA has a large down day that it’s going to impact me significantly EVEN if we remain above my breakeven principle level where I put my stop.
For example, if I held to the current price of $890, the notional size of the 20 shares I originally bought is now $17,800. This means when we have a 5% down day like today in my portfolio, I AM GOING TO FEEL IT.
Most hedge funds don’t like this TYPE of volatility in their portfolio so they will incrementally reduce the position to maintain a specific notional amount. When they do this, they are decreasing their exposure to upside in order to decrease the sensitivity of their portfolio to the volatility of NVDA 0.00%↑.
Do you begin to see that simply holding a position with stops above breakeven and accepting the swings pays you a HUGE amount? This is what holding a winner looks like. Within this, just keep moving the stop up to key levels you don’t think will get hit until your thesis changes.
As a result of holding a winner, it will become a larger and larger position in the portfolio. It is possible that the position becomes larger than 50% notional of your portfolio. Most people reduce the position size when this happens instead of just moving stops up incrementally to key levels that allow you to maintain exposure.
Extreme returns only come from taking inordinate amounts of volatility.
As a disclaimer, there are ways to systematize these principles with greater precision in a portfolio but this is the very basic idea. Do you see why the entire hedge fund industry underperforms and never puts up Soros-like returns? None of them like volatility. This provides an opportunity for people like me in my role to warehouse risk and take the volatility while ALWAYS protecting my principles.
Conclusion:
I will end with this, I manage money in a very different way than most of the industry. I couldn’t imagine doing it any other way. There is never a smooth linear path in my return distribution. Now you understand why: Extreme returns only come from taking inordinate amounts of volatility.
How I think about markets:
https://twitter.com/Globalflows/status/1646907973690888193
Asset Class Report: Bonds, Equities, Quant Signals
The macro views and asset class views I have laid are taking place as expected. Equities continue to melt up because nominal growth is elevated, liquidity is plentiful and earnings are accelerating. I noted this in the equity article (link) and most recent bond report (